Pythagoras Theorem

a square plus b square is equal to c square

I love Maths.

I love solving problems.

And I had always liked this chapter called "Heights and distances," and I actually saw it being used in real life today (for those who are wondering when you will actually use sin theta, cos theta in your life, just believe in math and keep solving problems, that day will present before you).

On the site today, markings were to be made to start the excavation for the foundation. Markings of the outer boundary of the house are to be plotted on the field. We had local people working on the site, and that means there is no theodolite or plane table or any sophisticated surveying equipment except a measuring tape.

Initial Boundary Marking with rope and steel rods

And this is when I saw people apply math without giving much thought to why they are doing what they are doing.

Initially, an approximate boundary of 33' x 34' was made by anchoring threads to the ground using steel bars on the four sides.

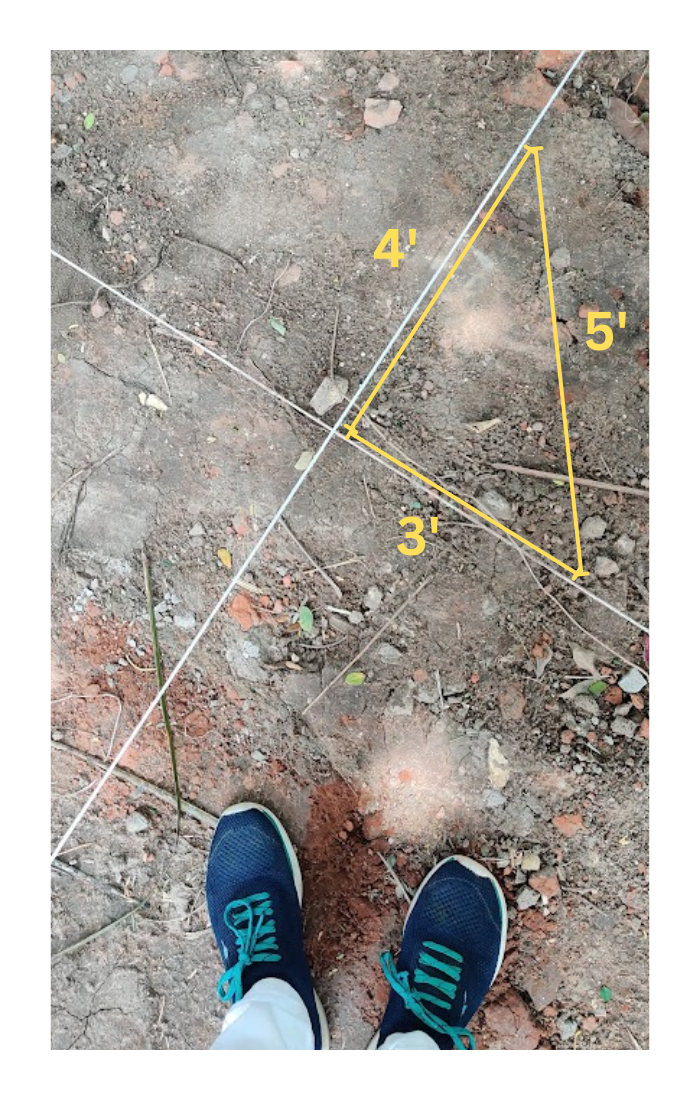
Once the approximate boundary was fixed, the right angles at the corners were to be checked, and for that, the head mason initially measured one side of the corner, made a marking on the thread at 4', and the adjacent perpendicular side was measured and marked at 3'. Now the distance between the marks was checked. If it was less than or greater than 5', adjustments were made by moving the string with its anchor a bit to the left or right. The trial and error were done until a perfect 3', 4', and 5' Pythagorean Triplet was achieved!

My inner self was proud of being able to assimilate the math that was being performed in front of my eyes.

One corner of the marking

Next comes the diagonals property for the rectangle. The mason Anna quickly asked me if I knew what should be the length between the opposite corners. I did a calculation on my mobile calculator, and it was 47.38 '. It was a struggle for him to figure out what I meant in decimals, and then I had to convert it to inches. (You have been taught your entire school and college life to convert everything to meters, centimetres, and millimetres, and on the site, you are forced to get used to feet and inches).

He verified if the distance between the diagonals matched exactly the value I mentioned.

He told me he wouldn't usually bother calculating the distances; instead, he would just measure the opposite diagonals and verify if they were equal. (Diagonals of a rectangle are equal - smart kid!)

This is the verification to see if all the corners are at perfect right angles.

Wow! How I wish when I learned these concepts in class, I got to do these exercises hands-on, and not a day would any of us forget what a^2 + b^2 is.

I bow before Pythagoras.

The world bows before Pythagoras!